Dynamic Range and Detectable Signal Change

The concept of defining the difference between Dynamic Range and Detectable Signal Change is important for understanding the limits of a detector in quantitative imaging. It is also beneficial for designing experiments based on the limits of the available detector and understanding when the detector or the signal itself is the limiting factor in the precision of the results.

Dynamic range of a detector is simplest concept. It is an easy calculation based on dividing the full well capacity of the detector by the noise of the detector. The full well capacity defines the maximum signal the detector can capture and the noise of the detector limits the smallest signal level that can be detected. This makes a simple ratio of maximum signal level to the noise of the detector, both expressed in electrons. This ratio does not tell us anything about the sensitivity to either high or low signal levels, just the ratio of one to the other.

Camera DR = FWC / N_c

Dynamic range in an image is similar to dynamic range in a detector but in this calculation the maximum signal level is used in place of the Full Well Capacity since the sample may not produce enough electrons to full the full well capacity. Detector noise is still the limit to the smallest signal level.

Image DR = S / N_c

Detectable Signal Change is also a simple concept but it has much more significance with regard to the precision of the data acquired with a detector. In this case we calculate the maximum possible detectable signal change based on the noise of the signal itself. Since photonic event signals have poisson distributions, the mean signal value will have a range of possible values that are different from the mean but not statistically different, based on the required precision of the measurement. Since the Full well capacity of the detector is the upper limit to the signal level, we can assume that if the detector is illuminated to the point at which it is just below saturation, then that is the maximum signal capability of the detector. Considering the poisson nature of light, we can estimate the noise of the signal itself by simply taking the square root of the detected signal. This is generally so much larger that the noise of the camera that the camera noise become irrelevant so our signal to noise calculation becomes just the square root of the signal level.

DSC = S / N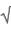_s = S

The importance of this is actually the percentage of the signal that is noise. This limits the precision of the measurement with no influence from the detector. It is obvious from this that as the signal decreases, the noise of the signal becomes a larger percentage of the signal. The detectable signal change is limited by the signal level itself.

% DSC = 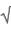S / S

The importance of the detectable signal change in quantitative applications is often overlooked. If an experiment is designed based on specific expectation of measuring a 1% difference in intensity, then the detector must have a least enough full well capacity to capture 10,000 electrons before saturating. If the expectation is 0.5 % detectable signal change, then the detector must have a minimum of 40,000 electrons full well capacity. In either case, the sample must produce enough photons to create that many electrons in the detector in the time allotted by the exposure and with the quantum efficiency of the system.

---